 Greatest Brillancy distance = 0.42 AU
Greatest Elongation distance = 0.69 AU
Greatest Brillancy distance = 0.42 AU
Greatest Elongation distance = 0.69 AU
Chapter 8 - Review Question #14 - Problems #7, #9
14. Explain why Mercury is never seen overhead at midnight in Earth's sky.
------------- Answer ------------------- Mercury is an inferior Planet with a semi-major axis of 0.39 AU - its maximum elongation from the Sun can only be at most 28 degrees. The Sun must be below the horizon to see Mercury - hence it will alway be less than 28 degrees altitude and alway near where the Sun sets or rises. Overhead = altitude of 90 degrees and at midnight the Sun is 90 degrees below the horizon that is an elongation of Mercury of 180 degrees which is impossible.
7. Compare the gravitational tidal effect of the Sun on Mercury with the tidal effect of Earth on the Moon (see Section 7.6).
------------- Answer ------------------- The differential tidal force is proportional to the Mass of the perturbing body and inversely proportional to the cube of its distance away. F ~ M/r3 M(sun) = 2x1030 kg ; M(Earth) = 6x1024 kg rSM = r(Sun-Mercury) = 0.39AU ; rEM = r(Earth-Moon) = 0.384/150 = 1/391 AU So.... F(Mercury)/F(Moon) = [M(sun)/M(Earth)[rEM/rSM)3 = (2x1030kg)/(6x1024kg)[1/(0.39x391)]3 = 0.094 The tidal forces of the Sun on Mercury are 1/10 of those of the Earth on the Moon.
9. Using the rate given in the text for the formation of 10-km craters on the Moon, estimate how long would be needed for the entire Moon to be covered with new craters of this size. How much higher must the cratering rate have been in the past to cover the entire lunar surface with such craters in the 4.6 billion years since the Moon formed?
------------- Answer ------------------- Crater Formation Rate: One new 10 km dia crater once every 10 million years = 10-7per year a - find the number of craters needed on the Moon - (Area of Moon)/(Area of Crater) b - Divide the number needed by the rate of formation to get the time period needed. Area of Crater ~ 10 kmx10 km = 100 km2 Radius of the Moon = 1738 km Area of the Moon = 4(pi)R2 = 4(3.14)(1738 km)2 = 3.80x107 km2 Number of Craters formed = 3.80x107 km2/100 km2 = 380,000 Time of Formation = 380,000/10-7per year = 3.8x1012 years = 3,800 Billion years The rate of formation to cover the Moon in 4.5 Billion years must be 3800/4.5 = 840 times faster than at present. ie. 840 per 10 million years or 84 10km dia craters per million year
Chapter 9 - Review Question #6, #9 - Problems #1, #10
6. Name three ways in which the atmosphere of Venus differs from that of Earth.
------------- Answer ------------------- Venus Earth ------- ------ 1 very dense less dense 2 Mostly CO2 Mostly N2 3 Little H20 Lot H20 4 No Oxygen Oxygen Atmosphere 5 50 km thick 10 km thick
9. Earth and Venus are nearly alike in size and density. What primary fact caused one planet to evolve as an oasis for life, while the other became a dry and inhospitable inferno?
------------- Answer ------------------- The Solar intensity at Venus' orbit is twice that at Earth's Orbit. The higher temperatures evaporated the water oceans and the UV from the Sun lead to the destruction of the water molecule. No water did not allow the formation of life.
1. Using the data given in the text, calculate Venus's angular diameter, as seen by an observer on Earth, when the planet is (a) at its brightest, (b) at greatest elongation, and (c) at the most distant point in its orbit.
------------- Answer -------------------
Angular Size(radians) = Diameter/Distance
Angular size("arc) = 206,300 Diameter/Distance
Use the diameter of Venus, its orbital radius and the orbital radius of the Earth.
diameter = 12104 km (1 AU = 150 million km)
= 8.07x10-5AU
a(Venus) = 0.723 AU
a(Earth) = 1.0 AU
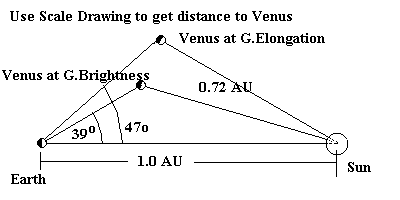
Note: The distance of Venus when it is brightest can be found by using the fact that the
elongation of Venus from the Sun at that time is 39 degrees. Likewise, at greatest elongation,
Venus has an elongation of 47 degrees and the distance can be found by trigonometry or a scale
drawing as above.
 Greatest Brillancy distance = 0.42 AU
Greatest Elongation distance = 0.69 AU
Greatest Brillancy distance = 0.42 AU
Greatest Elongation distance = 0.69 AU
| Position | Distance | Angular Size |
| Brightest | 0.42 AU | 40" arc |
| Greatest Elongation | 0.69 AU | 24" arc |
| Superior Conjunciton | 1.723 AU | 9.8" arc |
10. Calculate the orbital period of the Magellan spacecraft, moving around Venus on an elliptical orbit with a minimum altitude of 294 km and a maximum altitude of 8543 km above the planet's surface. In 1993 the spacecraft's orbit was changed to have minimum and maximum altitudes of 180 km and 541 km, respectively. What was the new period?
------------- Answer ------------------- To calculate the orbital period, we need the semi-major axis of the spacecraft. Radius of Venus = 6052 km rp = perivenus = 6052 + 294 = 6346 km ra = apvenus = 6052 + 8543 = 14,595 km a1 = (ra + rp)/2 = 10470 km The second Orbit has a2 = (180 + 541 + 12104)/2 = 6412 km Calculate the periods using Kepler's law P2 ~ a3/M Compare with a satellite around Earth. MVenus = 0.815 MEarth Use the Moon as a satellite of the Earth since we know its period as 27.3 days and its semi-major axis is 384,000 km P1 = PMoon [a1/aMoon]3/2 Mvenus/Mearth = 27.3 [10470/384000]3/2 (0.815) = 0.100 days = 2.4 hours P2 = PMoon [a2/aMoon]3/2 Mvenus/Mearth = 27.3 [6412/384000]3/2 (0.815) = 0.048 days = 1.15 hours
Project with ECU - Determine where Venus is with respect to the Sun (Elongation) on the following Dates: Nov 1, 1998, Dec 1, 1998, Jan 1, 1999, Feb 1, 1999, Mar 1, 1999 Describe where to look for Venus in those coming months and what it will look like (how bright and where).
| Date | Elongation from Sun | Magnitude | Sunset Position |
|---|---|---|---|
| Nov 1, 1998 | 1o NW | -3.9 | not visible |
| Dec 1, 1998 | 8o W | -3.9 | lost in twilight |
| Jan 1, 1999 | 15o W | -3.9 | SW very low |
| Feb 1, 1999 | 23o W | -3.9 | SW bright star |
| Mar 1, 1999 | 29o W | -4.0 | WSW bright star * |
* Note: Venus and Jupiter pass each other at only 0.1 deg at the end of February and Mercury is at
greatest elongation east at the first of March - a great time to observe planets at Sunset!!!
Sky at Sunset Mar 1, 1999
+ Saturn
+ Venus
+ Jupiter
+ Mercury
-----------------------W------------Horizon